A trebuchet is a type of catapult that converts the potential energy of a counterweight into the kinetic energy of a projectile. The simplest version operates like a see-saw, with the counterweight suspended from a hanger attached to the short arm and the projectile held in a sling attached to the throwing arm. When the short arm is raised and then released, the throwing arm rotates faster because it is longer and the sling rotates even faster as it whips around the end of the throwing arm.
At their inception during the Middle Ages, trebuchets were used to hurl boulders at or over castle walls in an attempt, usually successful, to batter them down. Modern trebuchet designers, lacking castles to besiege and fair maidens to rescue, must content themselves with hurling cooking pumpkins for distance. Competition, however, is fierce, and the winner of the contest can expect any fair maidens present at the pumpkin festival to hurl themselves at him. Thus, it behooves us to put as much study into the ballistics of pumpkins as old-time mathematicians put into the study of cannonballs.
Since trebuchets are the medieval version of mortars, let us first review what is known about mortars. Neville de Mestre (1990, p. 50) writes:
Frequently there are obstacles between a gun and the enemy, so weapons have been developed which enable the projectile to go over the obstacle and deliver a plunging fire on the enemy. These weapons are usually characterized by a low initial velocity and a high angle of departure, as for example with trench mortars. Since the velocity of the projectile is low its trajectory will not be very long and therefore cannot extend through a great depth of the atmosphere. Thus it is possible to assume constant atmospheric density and, as long as the projectile’s velocity is less than 240 ms-1, it is a reasonable approximation to take the drag to be proportional everywhere to the square of the speed. These approximations were first suggested by Euler (1707 – 1783).
These are also the basic assumptions which guide the analysis in this paper:
Axiom #1: Constant atmospheric density from the ground to the apogee.
Axiom #2: Drag is proportional everywhere to the square of the speed.
Axiom #3: Gravity is everywhere pointed downwards; e.g. the Earth is flat.
From wind tunnel tests we know that the coefficient of drag, CD, of a pumpkin-sized cannonball is 0.2. This is not bad, as smaller spheres, such as musket shot, have a CD of 0.45 due to the fact that their Reynolds number is below the critical point where the air flow in the boundary layer changes from a smooth motion to a turbulent one. Somewhat counter-intuitively, a turbulent boundary layer carries more energy and is thus able to remain in contact with the surface a bit longer. This narrows the wake and increases the pressure behind the sphere. The reduction in form resistance more than compensates for the increase in viscous resistance, so there is a net reduction in drag.
By experiment (in my kitchen sink) I determined that a 0.907 kg pumpkin floated with just over half of itself submerged under water. The same pumpkin, with 0.27 kg of water injected into its hollow core and the air removed, floated just barely submerged. Thus, pumpkins of this size have a specific gravity of 0.77 and their hollow core is capable of holding an additional 30% of their mass in water, which raises their specific gravity to 1.00. The tiny “Pee Wee” pumpkins are denser, with specific gravities near 1.00, and cannot be made much heavier with the addition of water. Specific gravity is the ratio of a pumpkin’s density to that of water, a cubic centimeter of which weighs one gram.
I filled my pumpkin with water by drilling a hole in it and then holding it under water overnight, being sure to position the hole on top. This can be accomplished more quickly with a large syringe, though one must be sure to make a vent for the air to escape. Large syringes are available from veterinarians who work with farm animals. Syringes are a somewhat restricted item so, if you are not obviously a farmer or a rancher, you can expect the veterinarian to question your need for a syringe. Hopefully, he will share your interest in ballistics.
In this paper, we will consider the ballistics of a pumpkin weighing 1.177 kg and having a diameter of 14.29 cm, which we will refer to as Pumpkin #1. We will compare its ballistics to that of a smaller pumpkin of diameter 13.10 cm that also weighs 1.177 kg due to having been filled with water. It will be referred to as Pumpkin #2. Because they are of the same weight, we can expect the trebuchet to launch them at the same angle and velocity.
Since everybody knows that the optimal launch angle is very close to 45°, we will here assume that the trebuchet designer has tuned his treb to launch pumpkins at this angle. Later, we will investigate the effect of launching pumpkins at other angles.
Velocity can easily be determined by setting the potential energy of the suspended counterweight to the kinetic energy of the flying pumpkin, with an allowance for energy lost to friction, twisting of the trebuchet frame, inertia of the arms and swaying of the counterweight. For study purposes, we will assume that 25% of the potential energy is thus lost. The exact amount does not really matter since we are comparing pumpkins, not trebuchets. A person with a less efficient treb will see the same ratio between pumpkins #1 and #2, though with slightly smaller distances than those predicted. Similarly, if he is launching uphill or into a headwind, the distances the pumpkins are thrown may be less than predicted, but their ratio, which is the principle result of this paper, will still be accurate.
Potential energy is given by mCWgh, with mCW the mass of the counterweight, 117.7 kg; g the acceleration due to gravity, 9.81 m/s; and h the height of the counterweight, 2.0 m. Kinetic energy is given by mPv02/2 with mP the mass of the pumpkin, 1.177 kg; and v0 the initial velocity in meters per second. Setting kinetic energy to 75% of potential energy, we get v02 = 1.5mCWgh/mP = 2943 m2/s2.
The ballistic coefficient, c, is given by mP/(CDd2) with the diameter of the pumpkin, d, in meters and the mass, mP, in kilos. Here d is the diameter of a perfect sphere with the same mass as the slightly squashed sphere that we actually have. It is about halfway between the height and the width of the pumpkin. Since rulers are calibrated in centimeters, it is easy to forget to convert the pumpkin’s diameter into meters, but neglecting this will throw the whole calculation off.
This equation gives the ballistic coefficient at sea level, which is the altitude taken for the following examples. My computer program adjusts c for altitude by multiplying it by the density of the air at sea level divided by the density at the launch site. Because of axiom #1, we are assuming constant atmospheric density from the ground to the apogee. In the professional version of my software, this assumption is dropped and the program will continuously recalculate the adjustment for air density throughout the projectile’s flight.
Note that the ballistic coefficients of bullets are normalized to a standard projectile. Ballistic coefficients expressed in this system, known as the G1 Model, are not directly comparable to the ballistic coefficients of pumpkins. For a direct comparison, consider a soccer ball, a slow-pitch softball, a baseball and a golf ball, which have ballistic coefficients of 43, 48, 68 and 126, respectively. Pumpkins #1 and #2 have ballistic coefficients of 288.2 and 342.9, respectively.
We will determine the ratio of the square of velocity to the ballistic coefficient for each pumpkin and then find another number and multiply it by the ballistic coefficient to get the range, r. Evaluating f(x) to get this intermediate result is beyond the scope of this paper. Fortunately, for the simple case of spherical projectiles, both de Mestre (1990, p. 63) and Daish (1972, p. 126) have provided it in tabular format. Their tables agree and I have written a computer program to estimate this function with a natural cubic spline.
| |
|
Pumpkin #1 |
Pumpkin #2 |
| |
v02 = 1.5mCWgh/mP |
2943 |
2943 |
| |
c = mP/(CDd2) |
288.2 |
342.9 |
| |
x = v02sin(2φ)/c |
10.21 |
8.58 |
| |
y = f(x) |
0.74 |
0.64 |
| |
r = yc |
214.6 m |
220.7 m |
Observe that, by axiom #2, drag is proportional everywhere to the square of the speed. It was known even in Euler’s day, 250 years ago, that this assumption holds true as long as the projectile’s velocity is less than 240 m/s. Indeed, √2943 = 54 m/s is far below this threshold where the basic theory gives way to the more complicated theories of bullets and artillery shells.
So we see that the smaller pumpkin weighted with water gets about 3% more range than an equally massive pumpkin of a larger size but with a hollow core. The fair maidens at the pumpkin festival will be all over you when you outdistance your rivals by a whole six meters!
Does 200 plus meters seem like an exaggeration for pumpkin hurling? Keep in mind that the range in a vacuum is given by v02/g. For v02 = 2943 m2/s2, this is 300 m. So pumpkins #1 and #2 have a 71.5% and a 73.6% ballistic efficiency, respectively. That air resistance should rob the pumpkin hurler of almost third of his range does not seem unreasonable.
Frankly, what really seems like an exaggeration is the typical hurler’s belief that his trebuchet is launching pumpkins at “about” 45°. While I have only been interested in trebuchet design for a few weeks, I have viewed a number of YouTube videos of various people’s trebs. By the simple expedient of holding a protractor up to my computer screen, I have observed that their launch angles are usually closer to 25°.
It is possible that people are not analyzing their own videos. Or, possibly, the fact that their projectiles are released well over their heads gives them the illusion that their projectile’s altitude is due to a steep angle rather than to initial height. But, whatever the cause of the problem, designers need to put more work into tuning their trebs to launch at 45° before committing their inventions to YouTube.
In the first draft of this paper, I wrote that everybody knows that the optimal launch angle is very close to 45°. I assumed that deviations from this ideal were inadvertent. However, after joining the Catmess (Catapult Message Board), I found that the majority of hurlers, or at least the most vocal ones, earnestly believe that 45° is “simplistic” and “nonsense.” For example, one guy, who has constructed a trebuchet that launches at 24°, writes:
Trig is something I never used and forgot around 1962. When working with trebs you need to divorce yourself from pure mathematics. The real world is very different.
Galileo proposed the 45° launch angle, and he was a bright guy, but he didn't know everything. Don't be too much in love with pure mathematics. The 45° thing may be valid for a parabolic flight path, but missiles don't follow parabolas except at low speeds and/or with high ballistic coefficients.
I've seen a claim that 31° was the optimum launch angle, and the claimant had math to back it up. You know what they say about figures don't lie.
Ron Toms, the forum administrator, concurs:
The interaction between the vertical and horizontal components of the trajectory interact differently at the beginning than at the end, and you need a lower angle to get the most distance. That 45° nonsense only works in a vacuum because the horizontal component must remain constant (no drag) for it to work out that way.
For comparison purposes, I will now find the range of Pumpkin #2 when launched at an angle of 24° or 31° instead 45°, as previously calculated. Note that 342.9 is not a particularly high ballistic coefficient; iron shot of the same diameter would be 2473.
| |
|
24° angle |
31° angle |
45° angle |
| |
v02 = 1.5mCWgh/mP |
2943 |
2943 |
2943 |
| |
c = mP/(CDd2) |
342.9 |
342.9 |
342.9 |
| |
x = v02sin(2φ)/c |
6.38 |
7.58 |
8.58 |
| |
y = f(x) |
0.51 |
0.58 |
0.64 |
| |
r = yc |
173.2 m |
200.5 m |
220.7 m |
Thus, we see that a pumpkin launched at a 24° angle has only 78% the range of one launched at a 45° angle. No fair maidens for you!
Full disclosure: The optimal angle is actually 43°, which achieves an additional 0.5 m. If one's treb is particularly tall and/or one is firing over a slight downgrade, one must lower one's angle of elevation another degree or two. The professional version of my software is capable of such accuracy, but the one that I was using when I wrote this paper is only approximate. I will stand by my assertion that 45° is the optimal launch angle until I meet someone who can calibrate his treb’s launch angle in one-degree increments.
C. B. Daish (1972, p. 51) writes:
For a cricket ball thrown at 30 meters per second in still air, the maximum range is achieved if it is projected at an angle of 42½° with the horizontal, but there is only a very slight variation, amounting to 30 cm or so, between the lengths of the throws over the range of angles between 40° and 45°. This variation is of no practical consequence at all.
The professional version of my software confirms Daish’s result. The optimal angle is 43°, but no angle between 40.8° and 45.1° is more than 0.5 m short of that. (The pumpkin is half a degree closer to 45° than the cricket ball because it has a slightly higher ballistic coefficient.) Really, this level of accuracy is not needed by trebuchet designers – my pumpkin program is sufficient. People adjust this angle by bending a wood screw with a pair of pliers, after all.
Frankly, I think Ron Toms knows that the optimal angle is very close to 45°. He sits idly by while amateur trebuchet designers earnestly debate whether the optimal angle is 24° or 31° and then, when I tell them that it is 45°, Toms jumps into the fray to denounce me in the most insulting language that he can think of. Why? Because he and his buddies are launching pumpkins at or near 45° while enjoying the adulation of amateurs who do not realize that they could do the same thing if they just dialed their launch angle back 20°. Trebuchet design is, as far as I know, Ron Toms’ only source of income and, when it comes to paying the rent, honor goes out the window.
Finally, I see no evidence that the opening of a nylon pouch imparts spin to pumpkins. Balls are given spin when they roll; for example, a baseball rolls off the pitcher’s fingertips or a golf ball rolls up the angled face of a club. A golf ball is violently struck by a steeply angled clubface made of unyielding steel. It accelerates from a standstill up to 60 to 70 m/s in 0.5 milliseconds, which is a very different thing than a pumpkin gently nestled in a smooth nylon pouch and taking a thousand times longer to accelerate up to 40 to 50 m/s, at which point the pouch just opens, without any twisting motion. Anyway, pumpkins lack the raised stitches or dimples that cause baseballs or golf balls to curve.
There is a group of guys at the Catmess who seem to think that the phrase “Magnus effect” is a sort of magical incantation that, when spoken, will dispel any doubts about the most outlandish braggadocio. These guys are probably amateur golfers who learned this big and very scientific-sounding word from their golf pro. Observing the rather unpredictable trajectories of their own golf balls at the driving range, they concluded that ballistics has a sort of anything-goes quality that is ideally suited to internet bragging.
For example, one fellow writes, “I have seen a pumpkin fired at a depressed angle (below horizon) which climbed to a 15° ascent and continued for about 2,000 feet.” When asked for an explanation of this gravity-defying feat, he replies, “Is it so hard to accept that a pumpkin may have sufficient spin imparted to it at 120+ mph with which to cause positive lift?”
Ron Toms concurs:
Go to any - once again, ANY - hurling competition and watch the pumpkins spin like crazy out of the pouch. Listen to the whistle of the bowling balls, and tell me that thing ain't spinning. I dare you.
According to Daish (1972, p. 62), a golf ball requires as much as 7800 rpm to overcome gravity.
The spins imparted to golf balls by the use of lofted clubs are surprisingly high… With a 5-iron, it is about 100 revolutions per second [6000 rpm], rising to 130 [7800 rpm] with a 7-iron.
Pitched baseballs rotate at up to 1600 rpm (Daish, 1972, p. 58) and do not have positive lift. A “rising fastball” just falls slower than we expect to see things fall and thus it creates the illusion of actually rising. Since misjudging the fall by even a few centimeters is the difference between a strike and a base hit, the effect of spin is important in baseball. But, if the pitcher were asked to throw for distance, this little bit of spin would be negligible and he would use the same 45° angle that a slingshot of the type used for fielding practice uses.
Since the throwing arm of a trebuchet rotates at no more than seventy rpm, it is indeed hard for this author to accept that it may impart on a pumpkin sufficient spin to cause positive lift.
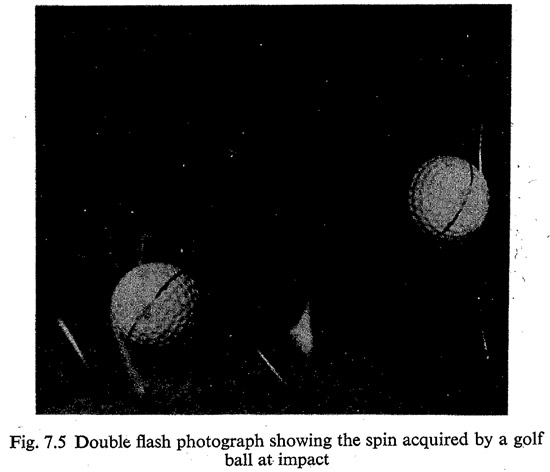
Daish (1972, p. 62) writes:
The photograph shows that, during this interval, the ball was rotated through an angle of about 180°, corresponding to a rate of spin of about 150 revolutions per second [9000 rpm].
If a car’s engine ran this fast, it would throw a rod. And Ron Toms would have us believe that “a pumpkin fired at a depressed angle (below the horizon) will climb to a 15° ascent and continue for about 2,000 feet?” Get real. A pumpkin is a vegetable. If it were possible to spin pumpkins this fast – which it is not – one would create instantaneous pumpkin pie.
Also, I might point out, all flying objects whistle, provided only that their Reynolds number is above the critical point where the air flow in the boundary layer changes from a smooth motion to a turbulent one. If I threw Ron Toms through the air at 120 mph, he’d whistle too. The tip of a sword can be heard whistling through the air. This noise has nothing to do with spin. The reason it is noticeable with trebuchet-launched projectiles is that, unlike mortars, there is no loud gunshot to distract the listener.
Obviously, there is not much that I can do to help these gentlemen. But, for those of you launching pumpkins at angles between zero and ninety degrees and with sling pouches that somehow manage to open without spinning the poor pumpkin up to thousands of rpm, my software will help you create a range table for your trebuchet.
Also, while I hesitate to impugn the craftsmanship that goes into these homemade devices, I must raise the possibility that they wobble. Perhaps sometimes their frames flex to the left and sometimes to the right. The resulting trajectories, being reminiscent of the hook or slice of a golf ball, conjured up talk of the Magnus effect, rather than a tightening of wood screws, which might have actually helped.
A wobbly treb is probably the explanation for this guy’s observations:
Turning sideways happened often to us; some went left, some went right, with same wind conditions. It all depends on projectile shape, center of gravity and the amount of spin the sling gives. There is no way to predict the way it will behave after release. That's the reason why I like hurling a lot. You never know what is gonna happen.
Frankly, I do not think I would want to stand too close to this guy's treb during a pumpkin launch. You never know what is gonna happen!
Filling a pumpkin’s hollow core with water makes it denser, which raises its ballistic coefficient and gives it more range. The larger a pumpkin, the more air space in its core and the more its density can be increased with the addition of water. But, the larger a pumpkin is, the slower it can be propelled by a given trebuchet. Because the effect of ballistic coefficient on range is proportional to the square of velocity, my advice is only relevant to people who consider a 15 cm pumpkin to be “small” and are capable of propelling more massive pumpkins at velocities over 50 m/s. Basically, if your treb is more suited to bowling balls than pumpkins, then filling pumpkins with water will help you set pumpkin-hurling records, though size restrictions will probably prevent you from actually entering any pumpkin-hurling contests.
Another advantage of adding water is that it gives pumpkins of every size a uniform specific gravity of 1.00, which makes target shooting easier. If different-sized spheres are all made of the same material, then their ballistic coefficient is given by πρd/(6CD) with ρ that material’s specific gravity and d their diameter in millimeters. The coefficient of drag, CD, is also constant, provided that the diameter does not change dramatically (causing the Reynolds number to pass the critical point) and the velocities are kept below Mach 0.6. Since trebuchet operators cannot adjust either counterweight mass or launch angle in the field, they must adjust for range by choosing the right-sized pumpkin, a task made much easier if all pumpkins have the same specific gravity, so their ballistic coefficient is a simple linear function of diameter.
My computer program will help you to determine the efficiency of your trebuchet and to devise a ballistic table that gives you the expected range for pumpkins of different weights.
Finally, I have dispelled two common myths about ballistics:
Myth #1
Ron Toms writes, “You need a lower angle to get the most distance. That 45° nonsense only works in a vacuum because the horizontal component must remain constant (no drag) for it to work out that way.”
I disagree. v2sin(2φ)/c is clearly at a maximum when φ = 45°. The optimal angle is slightly less but, for the velocities achieved by projectiles like baseballs and pumpkins, neither Neville de Mestre nor C. B. Daish found them measureable. For the example that I give in this paper, the optimal angle is actually 43°, which achieves an additional 0.5 m. The professional version of my software is capable of such accuracy, but the one that I was using when I wrote this paper is only an approximation. I will stand by my assertion that 45° is the optimal launch angle until I meet someone who can calibrate his treb’s launch angle in one-degree increments.
Myth #2
Ron Toms writes, “Go to any - once again, ANY - hurling competition and watch the pumpkins spin like crazy out of the pouch. Listen to the whistle of the bowling balls, and tell me that thing ain't spinning. I dare you.”
I disagree. A throwing arm rotating at seventy rpm could not possibly give a pumpkin the 7000 rpm it needs for positive lift. In fact, I see no evidence that it gives the pumpkin any spin. Balls spin because they roll and the opening of a smooth nylon pouch does not cause the pumpkin to roll off of the suddenly flaccid nylon. It just departs, with linear, but not angular, momentum. A week before Ron Toms made his comment about pumpkins spinning like crazy, I wrote, “If you have a large pumpkin and paint a green stripe on it, you could probably count the revolutions in a high-speed video.” But, instead of conducting this simple experiment, he dared me to meet him in person so we could brawl like teenagers instead of presenting evidence like scientists would.
REFERENCES
Daish, C. B. 1972. The Physics of Ball Games. London, UK: English University Press
de Mestre, Neville. 1990. The Mathematics of Projectiles in Sports. Cambridge, UK: Cambridge University Press